zoomanna.blogg.se

• #Sequences convergence to divergence series

We start by de ning sequences and follow by explaining convergence and divergence, bounded sequences, continuity, and subsequences. In this paper, we discuss the basic ideas involved in sequences and convergence. Here's a not-so-trivial example: $a_n=\cos(\pi n)\frac1=-1$, so those two have different limits: consequently $b_n$ cannot converge (in fact, it oscillates between $1$ and $-1$).Īs for your question «What's the trick to finding the sub-sequence?»: intuition. 1 If a n n is convergent, then there is some limit L to which it converges. INTRODUCTION TO THE CONVERGENCE OF SEQUENCES BECKY LYTLE Abstract. (Note that this limit I'm speaking of might as well be infinite.) One reason sequences are so useful is that humans often times have a discrete way of thinking. Thus if we wanted to prove a sequence is divergent and know it is.

#Sequences convergence to divergence series

Sequences allow us to take limits of discrete processes rather than those occuring over continuous time. The contrapositive of this statement says that a sequence is divergent if it is unbounded. Series, Convergence, Divergence Manipulating Absolutely Convergent Series Computing Series Partial Sums Infinite Series Basic Definition of Infinite Series.

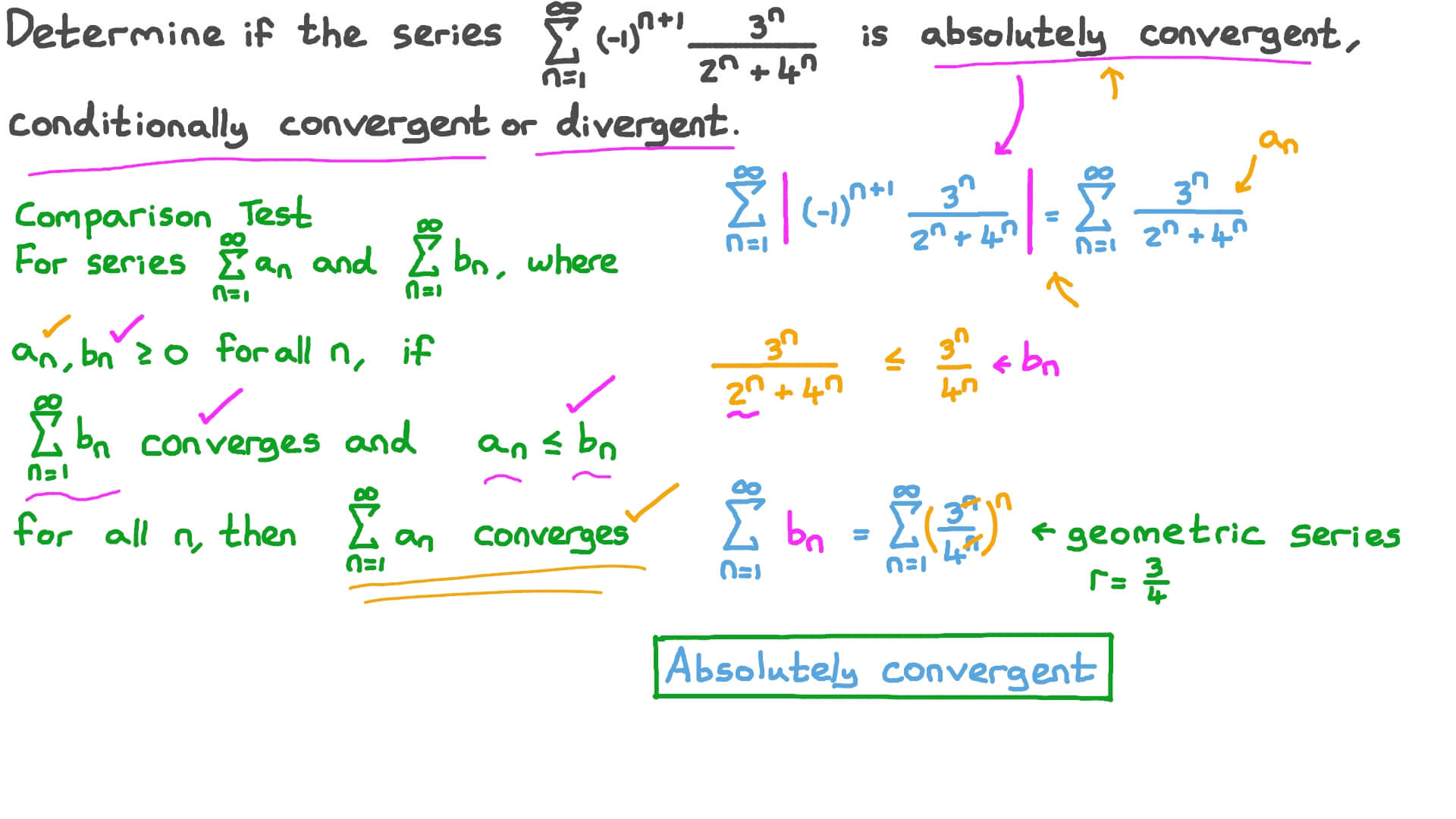
If that's not the case, then your sequence doesn't have a limit. BASICS OF SEQUENCE CONVERGENCE AND DIVERGENCE IAN MILLER 0.1. You can see by yourself that this can't be used to determine the convergence of a sequence: you just can't calculate an infinite amount of limits!Īnyway, if an easy subsequence you found converges to a certain limit, then you know what the overall limit should be. If you can't guess the limit of the whole sequence, you can start with a simpler subsequence: this is just a hunch, though: a sequence converges to a limit $\ell$ if and only if each of its subsequences converges to $\ell$ too. That explanation you gave with subsequences isn't usually the easiest (at least, as far as I know). An easy example of a convergent series is n112n12+14+18+116+ The partial sums look like 12,34,78,1516, and we can see that they get closer and closer to 1. Divergent series typically go to, go to, or dont approach one specific number. However, the series n1 to n(1/n) diverges toward infinity. For example, the sequence as n of n(1/n) converges to 1. There are many other criteria, though, you can use to prove it without calculating the sum. A divergent series is a series whose partial sums, by contrast, dont approach a limit. They can both converge or both diverge or the sequence can converge while the series diverge. These conditions that affect convergence or divergence may cause symptoms such as seeing double at near or far while viewing an object. If you're talking about series, directly calculating its limit is not, generally, the easiest way to prove it converges. Divergence excess is an over-divergence when attempting to look at distant objects.

it's given by a recursion formula, then you could use the so-called Cauchy condition: if the sequence lies in a complete space (such as $\mathbb R$, $\mathbb C$), it converges if and only if it satisfies the condition that for all $\epsilon>0$, there exists an $N\in\mathbb N$ such that for all $n, m>N$ you have $|a_n-a_m|<\epsilon$.

One of the following infinite series CONVERGES.

Estimate the value of a series by finding bounds on its remainder term. Use the integral test to determine the convergence of a series. There are two series that are important to know for a variety of reasons. Key Concepts Key Equations Glossary Learning Objectives Use the divergence test to determine whether a series converges or diverges.